A Case of Pre-FTC Definite Integral

August 28, 2017October 6, 2022 / Michael Xue / 4 Comments

Screen Shot 2017-08-29 at 9.23.39 PM.png

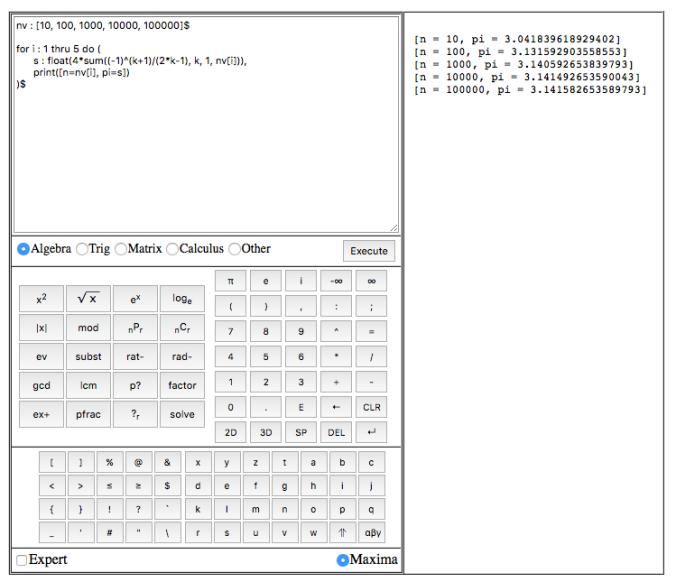
Fig. 1

By recalling the general formula for power summation derived in “Little Bird and a Recursive Generator“, namely

$\sum\limits_{i=1}^{n}{i^{p}} = \frac{n(n+1)^{p}- \sum\limits_{j=2}^{p}\binom{p}{j}\sum\limits_{i=1}^{n}i^{p-j+1}}{p+1}\quad\quad\quad(1)$

We can obtain the result

$\int\limits_{a}^{b}x^{p} dx = {{b^{p+1}-a^{p+1} }\over {p+1}}$

where $b\ge a\ge 0$ and $p \in Z_{0}^{+}$ , without the Fundamental Theorem of Calculus.

To this end, we divide the interval $[0, b]$ into $n$ sub-intervals of equal length as shown in Fig. 1. Let $s_{n}$ denotes the sum of the areas of the rectangles. The value $s_{n}$ tends to as $n$ increases, is the definite integral of $x^{p}$ from $x=0$ to $x=b$ , i.e.,

$\int\limits_{0}^{b} x^{p} dx = \lim\limits_{n \to \infty} s_{n}$ .

Since

$s_{n} = \sum\limits_{i=1}^{n-1}\frac{b}{n}(i\frac{b}{n})^p= \frac{b^{p+1}}{n^{p+1}}\sum\limits_{i=1}^{n-1}i^p$

It follows from (1) that

$s_{n} = \frac{b^{p+1}}{n^{p+1}}\cdot\frac{(n-1)n^{p}- \sum\limits_{j=2}^{p}\binom{p}{j}\sum\limits_{i=1}^{n-1}i^{p-j+1} }{p+1}$

$= \frac{b^{p+1}}{p+1}(\frac{(n-1)n^{p}}{n^{p+1}}-\frac{\sum\limits_{j=2}^{p}\binom{p}{j}\sum\limits_{i=1}^{n-1}i^{p-j+1}}{n^{p+1}})$

$= \frac{b^{p+1}}{p+1}((1-\frac{1}{n})-\sum\limits_{j=2}^{p}\binom{p}{j}\frac{\sum\limits_{i=1}^{n-1}i^{p-j+1}}{n^{p+1}})$ .

For $0 \leq p < 2, s_{n}$ reduces to

$s_{n} = \frac{b^{p+1}(1-\frac{1}{n})}{p+1}$ ,

and the result immediately follows:

$\int\limits_{0}^{b}x^{p} dx = \lim\limits_{n \to \infty} s_{n}=\lim \limits_{n \to \infty}\frac{b^{p+1}(1-\frac{1}{n})}{p+1}=\frac{b^{p+1}}{p+1}$ .

For $p \ge 2$ , we let

$t_{n} = \frac {\sum\limits_{i=1}^{n-1}i^{p-j+1}}{n^{p+1}}$ .

Clearly,

$0 < t_{n} < \frac{(n-1) n^{p-j+1}}{n^{p+1}}=\frac{n-1}{n^{j}}$ .

Since $j \ge 2$ ,

$\lim\limits_{n \to \infty}\frac{n-1}{n^j}=0$ .

i.e.,

$\lim\limits_{n \to \infty} t_{n} = 0$ .

Therefore,

$\int\limits_{0}^{b}x^{p} dx = \lim\limits_{n \to \infty} s_{n}=\lim\limits_{n \to \infty} \frac{b^{p+1}}{p+1}((1-\frac{1}{n})-\sum\limits_{j=2}^{p}\binom{p}{j}t_{n})$

$=\frac{b^{p+1}}{p+1}(\lim\limits_{n \to \infty}{(1-\frac{1}{n})}-\sum\limits_{j=2}^{p}\binom{p}{j}\lim\limits_{n \to \infty}{t_{n}})=\frac{b^{p+1}}{p+1}$ .

Applying this result to the area from $0$ to $a$ we have

$\int\limits_{0}^{a}x^{p} dx = \frac {a^{p+1}}{p+1}$ ,

and by subtraction of the areas,

$\int\limits_{a}^{b}x^{p} dx = \int\limits_{0}^{b}x^{p} dx -\int\limits_{0}^{a}x^{p} dx = \frac {b^{p+1}-a^{p+1}}{p+1}$ .

Pumpkin Pi

August 7, 2017October 20, 2021 / Michael Xue / 2 Comments

Fig. 1

Among many images of carved pumpkin, I like the one above (see Fig. 1) the most. It shows Leibniz’s formula for calculating the value of $\pi$ . Namely,

$\pi=4\sum\limits_{k=1}^{\infty}\frac{(-1)^{k+1}}{2k-1}$ .

To derive this formula, we begin with finding the derivative of $\arctan{x}$ :

Let $y = \arctan{x}$ , we have $x=\tan(y)$ , and

$\frac{d}{dx}x=\frac{d}{dx}\tan{y}=\frac{d}{dy}\tan{y}\frac{dy}{dx}=\sec^{2}{y}\frac{dy}{dx} = (1+\tan^{2}{y})\frac{dy}{dx}$

$= (1+x^{2})\frac{dy}{dx}$ .

Since $\frac{d}{dx}x=1$ ,

$\frac{d}{dx}\arctan{x}=\frac{1}{1+x^2}\quad\quad\quad(1)$

It follows that by (1) and the Fundamental Theorem of Calculus,

$\int\limits_{0}^{1}\frac{1}{1+x^2}dx = \arctan{x}\bigg|_{0}^{1}=\frac{\pi}{4}$

i.e.,

$\frac{\pi}{4} = \int\limits_{0}^{1}\frac{1}{1+x^2} dx\quad\quad\quad\quad\quad\quad(2)$

From carrying out polynomial long division, we observe

$\frac{1}{1+x^2} = 1 + \frac{-x^2}{1+x^2}$ ,

$\frac{1}{1+x^2} = 1 - x^2 + \frac{x^4}{1+x^2}$ ,

$\frac{1}{1+x^2} = 1 - x^2 + x^4 + \frac{-x^6}{1+x^2}$ ,

$\frac{1}{1+x^2} = 1 - x^2 + x^4 - x^6 + \frac{x^8}{1+x^2}$ .

It seems that

$\frac{1}{1+x^2} = \sum\limits_{k=1}^{n}{(-1)^{k+1}x^{2k-2}} + \frac{(-1)^{n} x^{2n}}{1+x^2}\quad\quad\quad\quad\quad\quad(3)$

Assuming (3) is true, we integrate it with respect to $x$ from 0 to 1,

$\int\limits_{0}^{1}\frac{1}{1+x^2}dx=\int\limits_{0}^{1}\sum\limits_{k=1}^{n}(-1)^{k+1}x^{2k-2} dx + \int\limits_{0}^{1}\frac{(-1)^{n} x^{2n}}{1+x^2}dx$

$= \sum\limits_{k=1}^{n}(-1)^{k+1}\int\limits_{0}^{1}x^{2k-2}dx +(-1)^{n}\int\limits_{0}^{1}\frac{x^{2n}}{1+x^2}dx$

$= \sum\limits_{k=1}^{n}(-1)^{k+1}\frac{x^{2k-1}}{2k-1}\bigg|_{0}^{1}+(-1)^{n}\int\limits_{0}^{1}\frac{x^{2n}}{1+x^2}dx$ .

As a result of integration, (2) becomes

$\frac{\pi}{4} = \sum\limits_{k=1}^{n}\frac{(-1)^{k+1}}{2k-1} + (-1)^{n}\int\limits_{0}^{1}\frac{x^{2n}}{1+x^2}dx$ ,

or,

$\frac{\pi}{4} - \sum\limits_{k=1}^{n}\frac{(-1)^{k+1}}{2k-1} =(-1)^{n}\int\limits_{0}^{1}\frac{x^{2n}}{1+x^2}dx$ .

Therefore,

$|\frac{\pi}{4} - \sum\limits_{k=1}^{n}\frac{(-1)^{k+1}}{2k-1}|=|(-1)^{n}\int\limits_{0}^{1}\frac{x^{2n}}{1+x^2}dx | = \int\limits_{0}^{1}\frac{x^{2n}}{1+x^2}dx < \int\limits_{0}^{1}x^{2n}dx$

$=\frac{x^{2n+1}}{2n+1}\bigg|_{0}^{1}= \frac{1}{2n+1}$ .

Moreover, $\forall \epsilon > 0$ , we obtain $n > \frac{1}{2}(\frac{1}{\epsilon}-1)$ through solving $\frac{1}{2n+1} < \epsilon$ . It means that $\forall \epsilon > 0, \exists n^*=\frac{1}{2}(\frac{1}{\epsilon}-1)$ such that for all $n > n^*, |\frac{\pi}{4} - \sum\limits_{k=1}^{n}\frac{(-1)^{k+1}}{2k-1}|<\epsilon$ , i.e.,

$\lim\limits_{n\to\infty}{ \sum\limits_{k=1}^{n}\frac{(-1)^{k+1}}{2k-1}}=\frac{\pi}{4}$ .

Thus

$\pi = 4 \sum\limits_{k=1}^{\infty}\frac{(-1)^{k+1}}{2k-1}\quad\quad\quad(4)$

The numerical value of $\pi$ is therefore approximated according to (4) by the partial sum

$4 \sum\limits_{k=1}^{n}\frac{(-1)^{k+1}}{2k-1}=4(1-\frac{1}{3}+\frac{1}{5}-\frac{1}{7}+\dots+(-1)^{n+1}\frac{1}{2n-1})\quad\quad\quad\quad(5)$

Its value converges to $\pi$ as $n$ increases.

However, (5) is by no means a practical way of finding the value of $\pi$ , since its convergence is so slow that many terms must be summed up before a reasonably accurate result emerges (see Fig. 2)

Screen Shot 2017-08-10 at 9.33.13 PM.png

Fig. 2

I doubt Leibniz has ever used his own formula to obtain the value of $\pi$ !

Let me leave you with an exercise: Prove (3)

“Chaplin or Leibniz ?” Revisit

August 1, 2017October 6, 2022 / Michael Xue / Leave a comment

Once the closed form of power summation is derived (see “Little Bird and a Recursive Generator“) namely

$s_{p} = 1^p+2^p+3^p+\dots+n^p=\frac{n(n+1)^p - \sum\limits_{j=2}^{p}\binom{p}{j} s_{p-j+1}}{p+1}, \quad\quad p, n \in N^{+},$

the validity of the inequality in “Chaplin or Leibniz ?” is readily shown:

$1^p+2^p+3^p+\dots+n^p = \frac{n(n+1)^p - \sum\limits_{j=2}^{p}\binom{p}{j} s_{p-j+1}}{p+1}$

$< \frac{(n+1)(n+1)^p - \sum\limits_{j=2}^{p}\binom{p}{j} s_{p-j+1}}{p+1}< \frac{(n+1)^{p+1}}{p+1}$ .

i.e.,

$(p+1)(1^p+2^p+3^p+\dots+n^p) < (n+1)^{p+1}, \quad\quad p, n \in N^+$ .