Does gravity matter ?

November 29, 2018June 23, 2022 / Michael Xue / Leave a comment

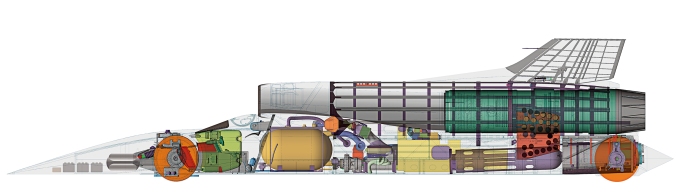
A single rocket expels its propellant at a constant rate $k$ .

Assuming constant gravity is the only external force, show that the equation of motion is

$(p+m_0-kt)\frac{dv}{dt}=ck-(p+m_0-kt)g$

where $v$ is the rocket’s speed, $c$ the speed of the propellant relative to the rocket, $p$ the payload mass, and $m_0$ the initial rocket mass.

If the rocket burn is continuous, show that the burn time is $\frac{\epsilon m_0}{k}$ and deduce that the final speed given to the payload is

$v=-c \log(1-\frac{\epsilon m_0}{m_0+p}) - \frac{g\epsilon m_0}{k}$

where $1-\epsilon$ is the structural factor of the rocket.

Estimate the percentage reduction in the predicted final speed due to the inclusion of the gravity term if

$\epsilon=0.8, \frac{p}{m_0}=\frac{1}{100}, c=3.0\;km\;s^{-1}, m_0=10^5\;kg$ , and $k=5 \times 10^3\;kg\;s^{-1}$ .

Find an expression for the height reached by the rocket during the burn and estimate its value using the data above.

Let’s recall the governing equation of rocket’s flight derived in “Viva Rocketry! Part 1“, namely,

$F = m\frac{dv}{dt} + u\frac{dm}{dt}$ .

In the present context, $m = m_0 + p- k t$ . It implies that

$\frac{dm}{dt}=-k$

and,

$F=-mg=-(m_0+p-kt)g$ .

With $u = c$ , we have

$-(p+m_0-kt)g = (p+m_0-kt)\frac{dv}{dt}+c\cdot(-k)$ ,

i.e.,

$(p+m_0-kt)\frac{dv}{dt}=ck-(p+m_0-kt)g$

$\frac{dv}{dt}=\frac{ck}{m_0+p-kt}-g$ .

The structural factor $1-\epsilon$ indicates the amount of fuel is $\epsilon m_0$ . Since the fuel is burnt at a constant rate $k$ , it must be true that at burnt out time $T$ ,

$\epsilon m_0 = kT$ .

Therefore,

$T=\frac{\epsilon m_0}{k}$ .

The solution to initial-value problem

$\begin{cases} \frac{dv}{dt}=\frac{ck}{m_0+p-kt}-g\\ v(0) = 0 \end{cases}$

tells the speed of the rocket during its flight while fuel is burnt (see Fig. 1):

$v = -c \log(1-\frac{kt}{m_0+p})-gt\quad\quad\quad(1)$

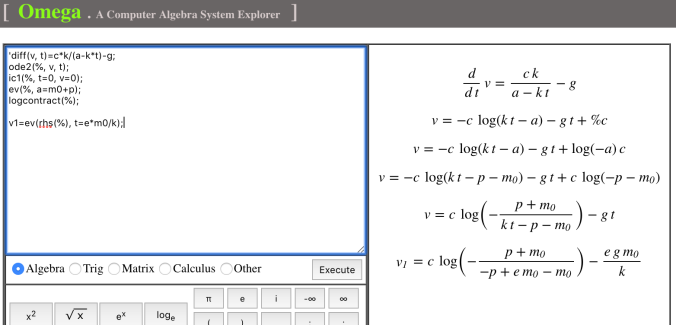
Screen Shot 2018-12-03 at 4.48.48 PM.png

Fig. 1

Evaluate (1) at burnt out time gives the final speed of the payload:

$v_1=-c \log(1-\frac{\epsilon m_0}{m_0+p}) - \frac{g\epsilon m_0}{k}\quad\quad\quad(2)$

Notice the first term of (2) is the burnt out velocity without gravity (see “Viva Rocketry! Part 1“)

It follows that the percentage reduction in the predicted final speed due to the inclusion of gravity is

$\frac{\frac{g \epsilon m_0} {k}}{-c \log(1-{\epsilon \over {1+\frac{p}{m_0}}})}\quad\quad\quad(3)$

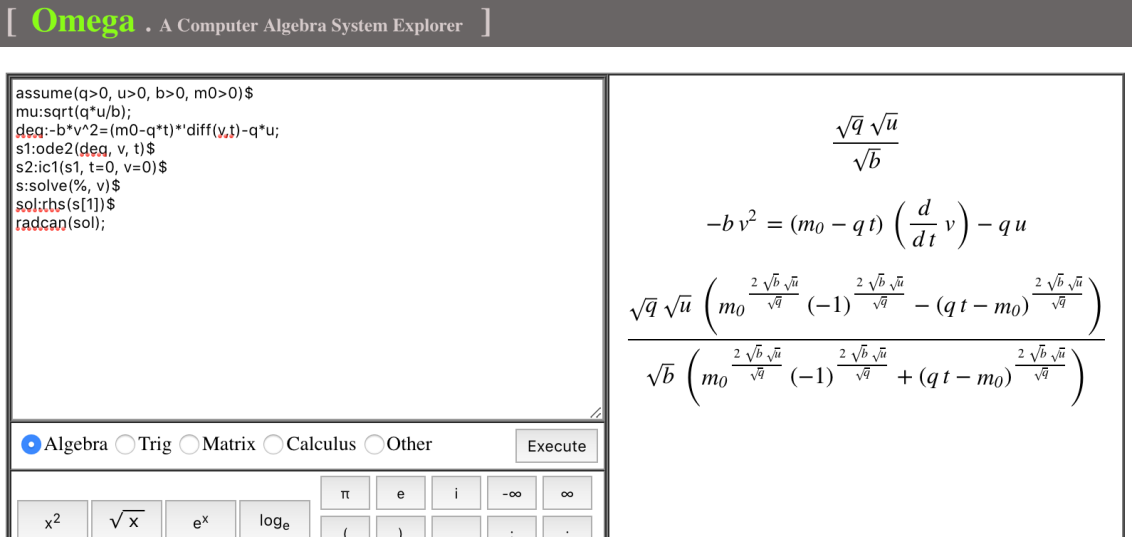
Using the given values which are typical, the estimated value of (3) (see Fig. 2) is

$0.003\%$ .

Screen Shot 2018-12-03 at 3.43.03 PM.png

Fig. 2

This shows the results obtained without taking gravity into consideration can be regarded as a reasonable approximation and the characteristics of rocket flight indicated in “Viva Rocketry! Part 1” are valid.

Since $v = \frac{dy}{dt}$ , (1) can be written as

$\frac{dy}{dt} = -c \log(1-\frac{kt}{m_0+p})-gt$

To find the distance travelled while the fuel is burnt, we solve yet another initial-value problem:

$\begin{cases}\frac{dy}{dt} = -c \log(1-\frac{kt}{m_0+p})-gt \\ y(0) = 0\end{cases}$

Screen Shot 2018-12-03 at 4.54.24 PM.png

Fig. 3

The solution (see Fig. 3) is

$y= -\frac{1}{2}g t^2 + ct - c\cdot (t-\frac{m_0+p}{k}) \cdot \log(1-\frac{kt}{m_0+p})$ .

Hence, the height reached at the burnt out time $t=\frac{\epsilon m_0}{k}$ is

$h = -\frac{g\epsilon^2 m_0^2}{2k^2}+\frac{c\epsilon m_0}{k}+\frac{c}{k}\cdot (p+(1-\epsilon)m_0) \cdot \log(1-\frac{\epsilon m_0}{m_0+p})$ .

Using the given values, we estimate that $h \approx 27 \; km$ (see Fig. 4)

Screen Shot 2018-12-03 at 3.34.13 PM.png

Fig. 4

Exercise 1: Find the distance the rocket travelled while the fuel is burnt by solving the following initial-value problem:

$\begin{cases}\frac{d^2y}{dt^2} =\frac{ck}{p+m_0-kt}-g \\ y(0) = 0, y'(0)=0 \end{cases}$

Thunderbolt

November 27, 2018October 7, 2022 / Michael Xue / Leave a comment

Fig. 1

Shown in Fig. 1 is an experimental car propelled by a rocket motor. The drag force (air resistance) is given by $R = \beta v^2$ . The initial mass of the car, which includes fuel of mass $m_f$ , is $m_0$ . The rocket motor is burning fuel at the rate of $q$ with an exhaust velocity of $u$ relative to the car. The car is at rest at $t=0$ . Show that the velocity of the car is given by, for $0 \leq t \leq T$ ,

$v(t) = \mu \cdot \frac{1-({m \over m_0})^{\frac{2\beta \mu}{q}}}{1+({m \over m_0})^{\frac{2\beta \mu}{q}}}$ ,

where $m=m_0-qt, \mu^2=\frac{qu}{\beta}$ , and $T=\frac{m_f}{q}$ is the time when the fuel is burnt out.

We have derived the governing equation of rocket flight in “Viva Rocketry! Part 1“, namely,

$F = m \frac{dv}{dt} + u \frac{dm}{dt}\quad\quad\quad(1)$

From $m=m_0-qt$ , we have

$\frac{dm}{dt} = -q$ .

Apply air resistance $R=\beta v^2$ as the external force, (1) becomes

$-\beta v^2 = (m_0 - q t) \frac{dv}{dt} - u q$ .

And the car is at rest initially implies

$v(0)=0$ .

It follows that the motion of the car can be modeled by an initial-value problem

$\begin{cases} -\beta v^2 = (m_0 - q t) \frac{dv}{dt} - u q \\ v(0) = 0 \end{cases}\quad\quad\quad(2)$

It suffices to show that the given $v(t)$ is the solution to this initial-value problem:

Screen Shot 2018-11-28 at 9.28.46 AM.png

Fig. 2

An alternative is obtaining the stated $v(t)$ through solving (2).

Screen Shot 2018-11-27 at 4.40.18 PM.png

Fig. 3

The fact that $m = m_0 -qt, (-1)^{2 \frac{\sqrt{b}\sqrt{u}}{\sqrt{q}}} = 1$ simplifies the result considerably,

$\frac{\sqrt q \sqrt u}{\sqrt \beta}\cdot \frac{m_0^{\frac{2 \sqrt \beta \sqrt u}{\sqrt q}}-m^{\frac{2 \sqrt \beta \sqrt u}{\sqrt q}}}{m_0^{\frac{2 \sqrt \beta \sqrt u}{\sqrt q}}+m^{\frac{2 \sqrt \beta \sqrt u}{\sqrt q}}}\quad\quad\quad(3)$

Divide both the numerator and denominator of (3) by $m_0^{\frac {2 \sqrt{\beta} \sqrt{u}}{\sqrt{q}}}$ then yields

$\frac{\sqrt q \sqrt u}{\sqrt \beta}\cdot \frac{1-(\frac{m}{m_0})^{\frac{2 \sqrt \beta \sqrt u}{\sqrt q}}}{1+(\frac{m}{m_0})^{\frac{2 \sqrt \beta \sqrt u}{\sqrt q}}}$

which is equivalent to the given $v(t)$ since $\mu^2=\frac{qu}{\beta}$ .

At time $t=T$ , the fuel is burnt out. It means

$m_0-m_f = m_0 - qT$ .

Therefore,

$T = \frac{m_f}{q}$

Exercise 1: Solve (2) manually.

Hint: The differential equation of (2) can be written as $\frac{1}{uq - \beta v^2} \frac{dv}{dt} = \frac{1}{m_0 - q t}$ .

Exercise 2: For $m_0=900\;kg, m_f=450\;kg, q=15\;kg/sec, u=500\;m/sec, \beta=0.3$ , what is the burnout velocity of the car?

Viva Rocketry! Part 1

November 10, 2018October 6, 2022 / Michael Xue / 7 Comments

In this post, we will first look at the main characteristics of rocket flight, and then examine the feasibility of launching a satellite as the payload of a rocket into an orbit above the earth.

A rocket accelerates itself by ejecting part of its mass with high velocity.

Screen Shot 2018-11-09 at 9.37.30 AM.png

Fig. 1

Fig. 1 shows a moving rocket. At time $t+\Delta t$ , the mass $\Delta m$ leaves the rocket in opposite direction. As a result, the rocket is being propelled away with an increased speed.

Let

$m(\square), m_{\square}$ – the mass of rocket at time ${\square}$

$\vec{v}_{\square}$ – the velocity of rocket at time $\square$

$v(\square), v_{\square}$ – the magnitude of $\vec{v}_{\square}$

$\vec{v}^*_{t+\Delta t}$ – the velocity of ejected mass $\Delta m$ at $t + \Delta t$

$v^*_{t+\Delta t}$ – the magnitude of $\vec{v}^*_{t+\Delta t}$

$u$ – the magnitude of $\Delta m$ ‘s velocity relative to the rocket when it is ejected. It is time invariant.

From Fig. 1, we have

$\Delta m = m_t - m_{t + \Delta t}$ ,

$\vec{v}_t = v_{t}$ ,

$\vec{v}_{t + \Delta t} = v_{t + \Delta t}$

and most notably, the relationship between $v^*_{t+\Delta t}, v_{t+\Delta t}$ and $u$ (see “A Thought Experiment on Velocities”):

$v^*_{t+\Delta t} = u - v_{t + \Delta t}$ .

It follows that

$\vec{v}^*_{t+\Delta t} = -v^*_{t+\Delta t} = v_{t + \Delta t} - u$ ,

momentum at time $t$ : $\vec{p}(t) = m_t \vec{v}_t = m_t v_t$

and,

momentum at time $t+\Delta t$ : $\vec{p}(t+\Delta t) = m_{t+\Delta t}\vec{v}_{t+\Delta t} + {\Delta m} \vec{v}^*_{t+\Delta t}=m_{t+\Delta t}\vec{v}_{t+\Delta t} + (m_t - m_{t+\Delta t}) \vec{v}^*_{t+\Delta t}$ $= m_{t+\Delta t}v_{t+\Delta t} + (m_t - m_{t+\Delta t})(v_{t+\Delta t}-u)$ .

Consequently, change of momentum in $\Delta t$ is $\vec{p}(t+\Delta t)- \vec{p}(t) = m_t (v_{t + \Delta t} - v_t) + u (m_{t + \Delta t} - m_t)$ .

Apply Newton’s second law of motion to the whole system,

$\vec{F}= {d \over dt} \vec{p}(t)$

$= \lim\limits_{\Delta t \rightarrow 0} {{\vec{p}(t+\Delta t) - \vec{p}(t)} \over \Delta t}$

$= \lim\limits_{\Delta t \rightarrow 0} { {m_t (v_{t + \Delta t} - v_t) + u (m_{t + \Delta t} - m_t)} \over {\Delta t} }$

$= \lim\limits_{\Delta t \rightarrow 0} {m_t {{v_{t + \Delta t} - v_t} \over {\Delta t}} + {u {{m_{t + \Delta t} - m_t} \over {\Delta t}}}}$

$= m_t \lim\limits_{\Delta t \rightarrow 0}{(v_{t+\Delta t} - v_t) \over {\Delta t}} + u \lim\limits_{\Delta t \rightarrow 0}{(m_{t +\Delta t} - m_t) \over \Delta t}$

That is,

$\vec{F} = m(t) {d \over dt} v(t) + u {d \over dt} m(t)$

where $\vec{F}$ is the sum of external forces acting on the system.

To get an overall picture of the rocket flight, we will neglect all external forces.

Without any external force, $\vec{F} = 0$ . Therefore

$0 = m(t) {d \over dt} v(t) + u {d \over dt} m(t)$

i.e.,

${d \over dt} v(t) = -{u \over m(t)} {d \over dt} m(t)\quad\quad\quad(1)$

The fact that $u, m(t)$ in (1) are positive quantities shows as the rocket loses mass ( ${d \over dt} m(t) < 0$ ), its velocity increases ( ${d \over dt} v(t) > 0$ )

Integrate (1) with respect to $t$ ,

$\int {d \over dt} v(t)\;dt = -u \int {1 \over m(t)} {d \over dt} m(t)\;dt$

gives

$v(t) = -u \log(m(t)) + c$

where $c$ is the constant of integration.

At $t = 0, v(0)=0, m(0) = m_1 + P$ where $m_1$ is the initial rocket mass (liquid or solid fuel + casing and instruments, exclude payload) and $P$ the payload.

It means $c = u \log(m_1+P)$ .

As a result,

$v(t) = -u \log(m(t)) + u \log(m_1+P)$

$= -u (\log(m(t) - \log(m_1+P))$

$= -u \log({m(t) \over m_1+P})$

i.e.,

$v(t) = -u \log(\frac{m(t)}{m_1+P})\quad\quad\quad(2)$

Since $m_1$ is divided into two parts, the initial fuel mass $\epsilon m_1 (0 < \epsilon < 1)$ , and the casing and instruments of mass $(1-\epsilon)m_1$ , $m(0)$ can be written as

$m(0) = \epsilon m_1 + ( 1 - \epsilon) m_1 + P$

When all the fuel has burnt out at $t_1$ ,

$m(t_1) = (1 - \epsilon)m_1 + P$

By (2), the rocket’s final speed at $t_1$

$v(t_1) = -u \log({m_1 \over {m_1+P}})$

$= -u \log({{(1-\epsilon)m_1+P} \over {m_1 + P}})$

$= -u \log({{m_1 + P -\epsilon m_1} \over {m_1+P}})$

$= -u \log(1-{{\epsilon m_1} \over {m_1+P}})$

$= -u \log(1-{\epsilon \over {1 + {P \over m_1}}})$

$= -u \log(1-{\epsilon \over {1 + \beta}})$

where $\beta = {P \over m_1}$ .

In other words,

$v(t_1) =-u \log(1-{\epsilon \over {1 + \beta}})\quad\quad\quad(3)$

Hence, the final speed depends on three parameters

$u, \epsilon$ and $\beta$

Typically,

$u = 3.0\;km\;s^{-1}, \epsilon = 0.8$ and $\beta = 1/100$ .

Using these values, (3) gives

Screen Shot 2018-11-05 at 9.21.14 PM.png

$v_1 = 4.7\;km\;s^{-1}\quad\quad\quad(4)$

This is an upper estimate to the typical final speed a single stage rocket can give to its payload. Neglected external forces such as gravity and air resistance would have reduced this speed.

With (4) in mind, let’s find out whether a satellite can be put into earth’s orbit as the payload of a single stage rocket.

We need to determine the speed that a satellite needs to have in order to stay in a circular orbit of height $h$ above the earth, as illustrated in Fig. 2.

Screen Shot 2018-11-08 at 3.47.09 PM.png

Fig. 2

By Newton’s inverse square law of attraction, The gravitational pull on satellite with mass $m_{s}$ is

${\gamma \; m_{s} M_{\oplus} \over (R_{\oplus} + h)^2}$

where universal gravitational constant $\gamma = 6.67 \times 10^{-11}$ , the earth’s mass $M_{\oplus} = 5.9722 \times 10^{24}\; kg$ , and the earth’s radius $R_{\oplus} = 6371\;km$ .